Lesson Plans:
Day 1:
Teacher will introduce students to what a prime and composite number is. To help understand the differences between numbers students will classify them into three categories: prime, composite, and square by looking at the factors of various number groups.
Students will be given manipulative blocks and asked to use their multiplication tables to set up the blocks in a manner that represent various multiplication problems. For example 10 x 10 = 100 and 50 x 2 = 100.
Using different color crayons students will identify the factors vs the product in their representation. Using the example above 10 x 10 = 100 and 50 x 2 = 100, students will determine the factors of 100. The factors are: 1, 2, 4, 5, 10, 20, 25, 50, and 100. These factors will be recorded on a sheet. Using the manipulative blocks allows students who are hands on learners to move the blocks around and break them apart as they determine the different factors.
Teacher will show students brain pop on "Prime Numbers" students will discuss characteristics of prime and composite numbers and record definitions and examples in their notes. Teacher will also remind students that the Commutative Property, while it may change the order of the numbers, it does not alter the final sum or product (ex: 50 x 2 = 100 and 2 x 50 = 100). Students will take Brain Pop quiz as a Ticket Out the Door (TOTD).
Day 2:
Students will review the differences between a prime and composite number, and the teacher will address misconceptions about prime and composite numbers discovered through yesterday's informal assessment.
- Prime numbers: Any number that has only two factors, 1 and itself (ex: 2 x 1 = 2)
- Composite numbers: numbers with more than two factors ( 8 x 1 = 8, and 8 can be broken into 4 x 2 = 8 or 2 x 2 x 2 = 8; factors of 8= 1, 2, 4, 8).
Prior to beginning the main lesson, the teacher will introduce the vocabulary word sieve to help draw upon students' prior knowledge. Students will be asked to think of something they might use around the house that would strain water (ex: when one makes mac and cheese). 
The strainer is called a sieve. Students will use a sieve to strain out prime and composite numbers from 1 to 100..
Teacher will introduce the Sieve of Eratosthenes to students through a SMART Board Lesson. Students will then practice the Sieve of Eratosthenes on their own.
----------------------------------------------------------------------------------------------------------------------------------
Sieve of Eratosthenes
To use the sieve of Eratosthenes to find the prime numbers up to 100, make a chart of the first one hundred positive integers (1-100):
1 2 3 4 5 6 7 8 9 10
11 12 13 14 15 16 17 18 19 20
21 22 23 24 25 26 27 28 29 30
31 32 33 34 35 36 37 38 39 40
41 42 43 44 45 46 47 48 49 50
51 52 53 54 55 56 57 58 59 60
61 62 63 64 65 66 67 68 69 70
71 72 73 74 75 76 77 78 79 80
81 82 83 84 85 86 87 88 89 90
91 92 93 94 95 96 97 98 99 100
- Cross out 1, because it is not prime.
- Circle 2, because it is the smallest positive even prime. Now cross out every multiple of 2; in other words, cross out every second number.
- Circle 3, the next prime. Then cross out all of the multiples of 3; in other words, every third number. Some, like 6, may have already been crossed out because they are multiples of 2.
- Circle the next open number, 5. Now cross out all of the multiples of 5, or every 5th number.
Continue doing this until all the numbers through 100 have either been circled or crossed out. You have just circled all the prime numbers from 1 to 100!
----------------------------------------------------------------------------------------------------------------------------------
To assess knowledge learned at the end of class students will write a "Dear Confused" Letter. In the letter students will tell Confused about the differences between prime and composite numbers. Students will also talk to Confused about how to use the Sieve of Eratosthenes to identify prime and composite numbers and what patterns were discovered through this activity. Lastly students will also talk to Confused about any questions they may still have about prime and composite numbers. Students will sign the letter Sincerely, (Their Name).
*** http://mathforum.org/dr.math/faq/faq.prime.num.html
Day 3:
Students will review their knowledge of factors by playing the Factor Game (created by Stacia from Collaboration Cuties Blog)
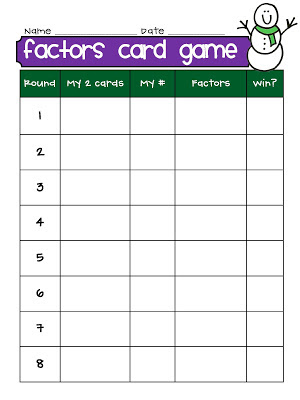How to play: Students work in pairs. Each student needs a recording sheet and a pencil, and each pair needs a set of playing cards. Students take turns picking two cards each round. They record their two cards on their recording sheet, and then they create a number using the cards. Students can put the cards together to create a number, add the cards, subtract the cards, multiply the cards, or divide the cards. For example, if I picked a 7 and a 9, I could make the number 79, 97, 16, 2, or 63. Then, once the students have created their numbers, they list all the factors for that number. Whoever has the most factors, wins! Whichever student wins the most rounds, wins the game!
*Teacher and students can also play this game using teams in the class. Breaking the class into teams of 4-5 or boys vs. girls.
Teacher will also review prime factorization and how students can use factor trees and/or the cake method to find the prime factorization of a number.
Cake Method: Prime Factors of 36 = 2, 2, 3, 3.
Factor Trees
Students will complete a TOTD answering 3 questions to help communicate their understanding of prime factorization with the teacher.
Day 4:
Students will review the Sieve of Eratosthenes from Day 2. Looking at the Sieve the teacher and students will identify what numbers marked are prime and which ones are composites. Using paper divided into three columns (prime, composite, and square) students will list the numbers from the chart that are prime, composite.
Teacher will introduce square numbers to students. Students will watch BrainPop on square numbers and answer assessment questions at the end of the clip. Afterwards students will pair off into groups and explore several multimedia websites including http://www.mathsisfun.com/square-root.html. Students will explore the characteristics of square numbers and which numbers would be an example of a square number.
Students will complete Prove or Disprove worksheet adapted from Utah Board of Education. Students will be given a variety of statements about prime, composite, and square numbers. Students must prove if the statements given are true or disprove if the statement given is false write a mathematical reason for their decision.
Day 5:
Students will spend class period today creating a Prezi or PowerPoint Presentation. On their multimedia project students will define prime, composite, and square numbers in their own words and how individuals determine if a number is prime, composite, or neither. In addition, students will identify what prime factorization is and explain how to factor a number to identify its prime factors. Students will also be given a variety of multimedia resources, class notes, and texts to use to help develop their final presentation. In addition, students will discuss how to use the Sieve of Eratosthenes to break the code in determining if a number is prime or composite. This assessment will count as a performance task grade.
Assessments:
Prior to beginning the unit, students will be given a pre-test. This pre-test will allow me to identify students' prior knowledge of the topic of prime, composite, and square numbers. In addition, I can use the assessment to ability group students for different activities. Vocabulary, even if math, is essential for students to understand. Therefore, it is important that time is spent ensuring students have a good understanding of the various vocabulary terms in the unit.
Students will also be assessed through many quizzes and informal assessments like tickets out the door and the Dear Confused Letter. These informal assessments provide me with an idea of what information the students are comprehending, as well as the misconceptions they have developed that I need to go back and address and reteach. One of the best informal assessments to use in any unit, that is orally given are students' questions. The level of questioning by the teacher and students' questions and responses can provide a good idea of students' thought process.
The post-test given to students will include a variety of question types: multiple choice, prove or disprove statements, and basic response questions. The pre- and post test will have similar type of questions to help the teacher identify the growth and understanding of students' knowledge towards reaching the learning goals and the unit objectives
----------------------------------------------------------------------------------------------------------------------------------
Prime,
Composite, and Square Numbers Assessment
Determine
whether each number is prime or composite.
1.
35 2. 55 3. 101 4. 2 5. 27 6. 17
Factor
each number complete. You may use a factor tree or the cake method. You must
show your work for credit.
7.
38 8. 56 9. 333 10.
81 11. 1234
Answer
the following questions in complete sentences. The more information you provide
the better.
12.
Explain why the number 0 and 1 are not considered prime numbers.
13.
How is a prime number different from a composite number?
Prove
or disprove the following statements. Include mathematical reasoning to support
your answer.
14.
There are 25 prime numbers between 1 and 100 and 25 prime numbers between 100
and 200. Is this true? Why or why not?
15.
Only three of the numbers below are prime numbers
123
453 587
198 233 267 307 7101
16.
What are square numbers?
17.
Which of the following would be a square number?
- 45
- 9
- 233
- 57
18.
Which best describes the square root of 16
- 4 x4
- 8 x 2
- 2 x 2 x 2 x 2
- 16 x 1
19.
Why are numbers 25, 49 and 64 known as perfect squares?
20.
Find the square root of 81.
Bonus:
Explain what patterns were discovered when you did the Sieve of Eratosthenes
activity.
